
MATH 3100 • Introduction to Probability

Noncolliding Macdonald walks with an absorbing wall
The branching rule is one of the most fundamental properties of the Macdonald symmetric polynomials. It expresses a Macdonald polynomial as a nonnegative linear combination of Macdonald polynomials with smaller number of variables. Taking a limit of the branching rule under the principal specialization when the number of variables goes to infinity, we obtain a Markov chain of $m$ noncolliding particles with negative drift and an absorbing wall at zero. The chain depends on the Macdonald parameters $(q,t)$ and may be viewed as a discrete deformation of the Dyson Brownian motion. The trajectory of the Markov chain is equivalent to a certain Gibbs ensemble of plane partitions with an arbitrary cascade front wall.
In the Jack limit $q=t^{\beta/2}\to1$ the absorbing wall disappears, and the Macdonald noncolliding walks turn into the $\beta$-noncolliding random walks studied by Huang [arXiv:1708.07115]. Taking $q=0$ (Hall-Littlewood degeneration) and further sending $t\to 1$, we obtain a continuous time particle system on $\mathbb{Z}_{\ge0}$ with inhomogeneous jump rates and absorbing wall at zero.
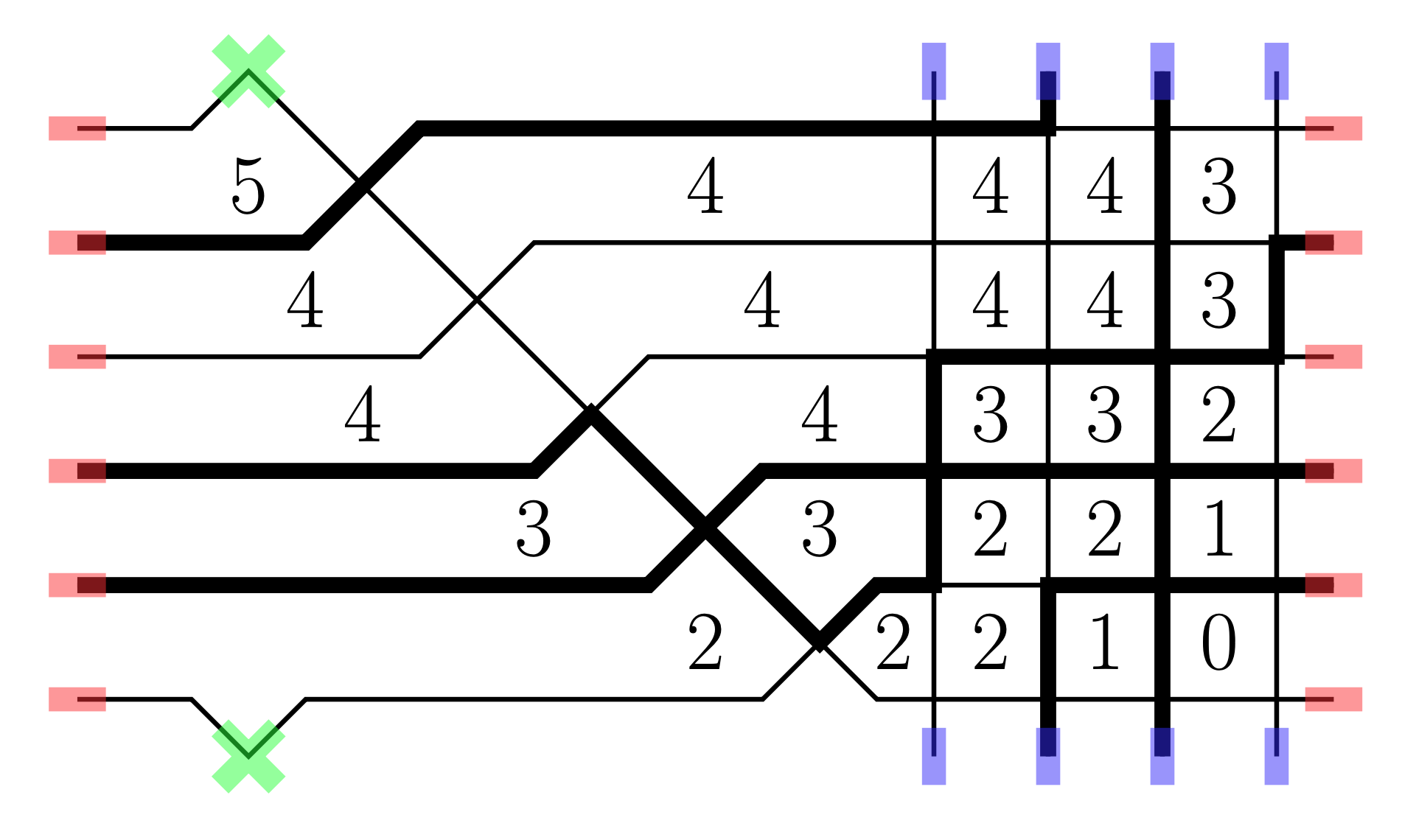
Irreversible Markov Dynamics and Hydrodynamics for KPZ States in the Stochastic Six Vertex Model
We introduce a family of Markov growth processes on discrete height functions defined on the 2-dimensional square lattice. Each height function corresponds to a configuration of the six vertex model on the infinite square lattice. We focus on the stochastic six vertex model corresponding to a particular two-parameter family of weights within the ferroelectric regime. It is believed (and partially proven, see Aggarwal, arXiv:2004.13272) that the stochastic six vertex model displays nontrivial pure (i.e., translation invariant and ergodic) Gibbs states of two types, KPZ and liquid. These phases have very different long-range correlation structure. The Markov processes we construct preserve the KPZ pure states in the full plane. We also show that the same processes put on the torus preserve arbitrary Gibbs measures for generic six vertex weights (not necessarily in the ferroelectric regime).
Our dynamics arise naturally from the Yang-Baxter equation for the six vertex model via its bijectivisation, a technique first used in Bufetov-Petrov (arXiv:1712.04584). The dynamics we construct are irreversible; in particular, the height function has a nonzero average drift. In each KPZ pure state, we explicitly compute the average drift (also known as the current) as a function of the slope. We use this to analyze the hydrodynamics of a non-stationary version of our process acting on quarter plane stochastic six vertex configurations. The fixed-time limit shapes in the quarter plane model were obtained in Borodin-Corwin-Gorin (arXiv:1407.6729).

MATH 3100 • Introduction to Probability (3 sections)

Inhomogeneous Interacting Particle Systems
I will survey results on stochastic interacting particle systems (such as TASEP, the Totally Asymmetric Simple Exclusion Process) in the presence of inhomogeneity. That is, we consider particles with variable speeds, or the space in which the system evolves contains “swamps and highways”, where particles move with variable speed. Inhomogeneity inserts multiple parameters into the system, but in some miraculous cases the system stays exactly solvable in their presence. This allows to observe interesting asymptotic phase transitions. Moreover, permutations of the multiple parameters can sometimes be realized as Markov operators on the state of the system, leading to intriguing structural properties.

Schur rational functions, vertex models, and random tilings
Discusses symmetric functions and stochastic models based on the free fermionic six vertex model. Follows joint work with Aggarwal, Borodin, and Wheeler.
The work deals with symmetric rational functions and probabilistic models based on the fully inhomogeneous six vertex (ice type) model satisfying the free fermion condition. Two families of symmetric rational functions $F_\lambda,G_\lambda$ are defined as certain partition functions of the six vertex model, with variables corresponding to row rapidities, and the labeling signatures $\lambda=(\lambda_1\ge \ldots\ge \lambda_N)\in \mathbb{Z}^N$ encoding boundary conditions. These symmetric functions generalize Schur symmetric polynomials, as well as some of their variations, such as factorial and supersymmetric Schur polynomials. Cauchy type summation identities for $F_\lambda,G_\lambda$ and their skew counterparts follow from the Yang–Baxter equation. Using algebraic Bethe Ansatz, we obtain a double alternant type formula for $F_\lambda$ and a Sergeev–Pragacz type formula for $G_\lambda$.
In the spirit of the theory of Schur processes, we define probability measures on sequences of signatures with probability weights proportional to products of our symmetric functions. We show that these measures can be viewed as determinantal point processes, and we express their correlation kernels in a double contour integral form. We present two proofs: The first is a direct computation of Eynard–Mehta type, and the second uses non-standard, inhomogeneous versions of fermionic operators in a Fock space coming from the algebraic Bethe Ansatz for the six vertex model.
We also interpret our determinantal processes as random domino tilings of a half-strip with inhomogeneous domino weights. In the bulk, we show that the lattice asymptotic behavior of such domino tilings is described by a new determinantal point process on $\mathbb{Z}^{2}$, which can be viewed as an doubly-inhomogeneous generalization of the extended discrete sine process.

Free Fermion Six Vertex Model: Symmetric Functions and Random Domino Tilings
Our work deals with symmetric rational functions and probabilistic models based on the fully inhomogeneous six vertex (ice type) model satisfying the free fermion condition. Two families of symmetric rational functions $F_\lambda,G_\lambda$ are defined as certain partition functions of the six vertex model, with variables corresponding to row rapidities, and the labeling signatures $\lambda=(\lambda_1\ge \ldots\ge \lambda_N)\in \mathbb{Z}^N$ encoding boundary conditions. These symmetric functions generalize Schur symmetric polynomials, as well as some of their variations, such as factorial and supersymmetric Schur polynomials. Cauchy type summation identities for $F_\lambda,G_\lambda$ and their skew counterparts follow from the Yang–Baxter equation. Using algebraic Bethe Ansatz, we obtain a double alternant type formula for $F_\lambda$ and a Sergeev–Pragacz type formula for $G_\lambda$.
In the spirit of the theory of Schur processes, we define probability measures on sequences of signatures with probability weights proportional to products of our symmetric functions. We show that these measures can be viewed as determinantal point processes, and we express their correlation kernels in a double contour integral form. We present two proofs: The first is a direct computation of Eynard–Mehta type, and the second uses non-standard, inhomogeneous versions of fermionic operators in a Fock space coming from the algebraic Bethe Ansatz for the six vertex model.
We also interpret our determinantal processes as random domino tilings of a half-strip with inhomogeneous domino weights. In the bulk, we show that the lattice asymptotic behavior of such domino tilings is described by a new determinantal point process on $\mathbb{Z}^{2}$, which can be viewed as an doubly-inhomogeneous generalization of the extended discrete sine process.